[Published online Journal of Computer Chemistry, Japan -International Edition Vol.8, -, by J-STAGE]
<Title:> Calculus of Several Harmonic Functions
<Author(s):> Kazuhiro ISHIDA
<Corresponding author E-Mill:> k-ishida(at)fancy.ocn.ne.jp
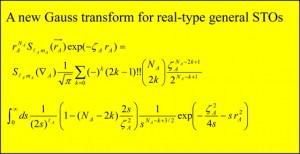
<Abstract:> Abstract: We describe several harmonic functions; the solid harmonics (SH), the mixed SH, the reducing SH and the reducing mixed SH. We do the solid harmonic gradient (SHG) operator. Using the SHG, we derive a new Gauss-transform and a new Fourier-transform for real-type general Slater-type orbitals (STO). Several formulas can be derived for the calculus of harmonic functions. They will be useful especially for evaluating the angular part of molecular integrals over STOs and over Gaussian-type orbitals with higher-angular-momenta.Keywords: Several harmonic functions; Molecular integrals; Solid harmonics; Higher-angular-momenta; Slater-type orbital; Solid harmonic gradient.
<Keywords:>
<URL:> https://www.jstage.jst.go.jp/article/jccjie/8/0/8_2021-0029/_html
<Title:> Calculus of Several Harmonic Functions
<Author(s):> Kazuhiro ISHIDA
<Corresponding author E-Mill:> k-ishida(at)fancy.ocn.ne.jp
<Abstract:> Abstract: We describe several harmonic functions; the solid harmonics (SH), the mixed SH, the reducing SH and the reducing mixed SH. We do the solid harmonic gradient (SHG) operator. Using the SHG, we derive a new Gauss-transform and a new Fourier-transform for real-type general Slater-type orbitals (STO). Several formulas can be derived for the calculus of harmonic functions. They will be useful especially for evaluating the angular part of molecular integrals over STOs and over Gaussian-type orbitals with higher-angular-momenta.Keywords: Several harmonic functions; Molecular integrals; Solid harmonics; Higher-angular-momenta; Slater-type orbital; Solid harmonic gradient.
<Keywords:>
<URL:> https://www.jstage.jst.go.jp/article/jccjie/8/0/8_2021-0029/_html