[Published online Journal of Computer Chemistry, Japan Vol.23, 13-18, by J-STAGE]
<Title:> New LCAO-MO Method for Molecules in The Arbitrary Magnetic Field
<Author(s):> Katsumi NAKAGAWA
<Corresponding author E-Mill:> hbo59418(at)kwansei.ac.jp
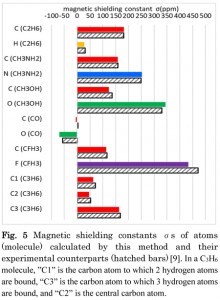
<Abstract:> The Hartree-Fock-Slater (HFS) equation including magnetic interaction was solved full-numerically by the LCAO-MO method. Firstly, the AOs of each atom in a molecule were obtained by solving the HFS equation dedicated to the atom. This equation includes the same magnetic interaction as the equation of the molecule and virtual “well potential” to adjust the spreads of AOs. In this method, the Fock operator for the atom is expressed as a numerical matrix and the equation can be solved by a mathematical solver of the numerical eigenvalue problem. Then an MO is obtained by using these AOs as bases of the LCAO-MO method. AOs naturally have suitable complex phases for the MO to satisfy gauge invariance. To evaluate the new method, magnetic shielding constants σ were calculated for various small molecules comprising 2-3 atoms of second-row element and hydrogen atoms. The “well potential” of each atom was adjusted systematically and calculated σ showed fairly good agreement with experimental values.
<Keywords:> LCAO-MO, magnetic field, DV-Xα, well potential, complex phase, gauge invariance, magnetic shielding constant
<URL:> https://www.jstage.jst.go.jp/article/jccj/23/1/23_2024-0002/_article/-char/ja/
<Title:> New LCAO-MO Method for Molecules in The Arbitrary Magnetic Field
<Author(s):> Katsumi NAKAGAWA
<Corresponding author E-Mill:> hbo59418(at)kwansei.ac.jp
<Abstract:> The Hartree-Fock-Slater (HFS) equation including magnetic interaction was solved full-numerically by the LCAO-MO method. Firstly, the AOs of each atom in a molecule were obtained by solving the HFS equation dedicated to the atom. This equation includes the same magnetic interaction as the equation of the molecule and virtual “well potential” to adjust the spreads of AOs. In this method, the Fock operator for the atom is expressed as a numerical matrix and the equation can be solved by a mathematical solver of the numerical eigenvalue problem. Then an MO is obtained by using these AOs as bases of the LCAO-MO method. AOs naturally have suitable complex phases for the MO to satisfy gauge invariance. To evaluate the new method, magnetic shielding constants σ were calculated for various small molecules comprising 2-3 atoms of second-row element and hydrogen atoms. The “well potential” of each atom was adjusted systematically and calculated σ showed fairly good agreement with experimental values.
<Keywords:> LCAO-MO, magnetic field, DV-Xα, well potential, complex phase, gauge invariance, magnetic shielding constant
<URL:> https://www.jstage.jst.go.jp/article/jccj/23/1/23_2024-0002/_article/-char/ja/